Solvmanifold

In mathematics, a solvmanifold is a homogeneous space of a connected solvable Lie group. It may also be characterized as a quotient of a connected solvable Lie group by a closed subgroup. (Some authors also require that the Lie group be simply-connected, or that the quotient be compact.) A special class of solvmanifolds, nilmanifolds, was introduced by Malcev, who proved first structural theorems. Properties of general solvmanifolds are similar, but somewhat more complicated.

Contents 1 Examples 2 Properties 3 Odd section 4 References

Examples

• A solvable Lie group is trivially a solvmanifold.

• Every nilpotent group is solvable, therefore, every nilmanifold is a solvmanifold. This class of examples includes n-dimensional tori and the quotient of the 3-dimensional real Heisenberg group by its integral Heisenberg subgroup.

• The Möbius band and the Klein bottle are solvmanifolds that are not nilmanifolds.

• The mapping torus of an Anosov diffeomorphism of the n-torus is a solvmanifold. For n=2, these manifolds belong to Sol, one of the eight Thurston geometries.

Properties

• A solvmanifold is diffeomorphic to the total space of a vector bundle over some compact solvmanifold. This statement was conjectured by G. Mostow and proved by L. Auslander and R. Tolimieri.

• The fundamental group of an arbitrary solvmanifold is policyclic.

• A compact solvmanifold is determined up to diffeomorphism by its fundamental group.

• Fundamental groups of compact solvmanifolds may be characterized as group extensions of free abelian groups of finite rank by finitely generated torsion-free nilpotent groups.

• Every solvmanifold is aspherical. Among all compact homogeneous spaces, solvmanifolds may be characterized by the properties of being aspherical and having a solvable fundamental group.

Odd section

Let 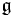be a real Lie algebra. It is called a complete Lie algebra if each map

ad

in its adjoint representation is hyperbolic, i.e. has real eigenvalues. Let G be a solvable Lie group whose Lie algebra 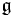is complete. Then for any closed subgroup Γ of G, the solvmanifold G/Γ is a complete solvmanifold.

References

• L. Auslander, An exposition of the structure of solvmanifolds I, II, Bull. Amer. Math. Soc., 79:2 (1973), pp. 227–261, 262–285
• Cooper, Daryl; Scharlemann, Martin (1999), The structure of a solvmanifold's Heegaard splittings, "Proceedings of 6th Gökova Geometry-Topology Conference", Turkish Journal of Mathematics 23 (1): 1–18, ISSN 1300-0098, MR1701636, http://mistug.tubitak.gov.tr/bdyim/toc.php?dergi=mat&yilsayi=1999/1 

• V.V. Gorbatsevich (2001), "Solvmanifold", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1556080104, http://www.encyclopediaofmath.org/index.php?title=S/s086100